A reminder will be sent out for each meeting. To subscribe to receive these reminders please send an email with the subject 'subscribe' to spip.meetings@gmail.com.
To volunteer to give a talk please email spip.meetings@gmail.com.
Previous talks can be found here.
Charles-Edouard Bréhier (Claude Bernard University Lyon 1) - Efficient sampling for the invariant distributions of SPDEs
October 30, 2019
When: 10:00
Where: F1.15 (KdVI)
We consider the question of sampling the invariant distribution of some ergodic (semilinear parabolic SPDEs) of the type du(t)=Au(t)dt+F(u(t))dt+dW(t), driven by space-time white noise.
A standard integrator is the linear implicit Euler scheme, however its weak order of convergence is only equal to 1/2 (contrary to 1 in finite dimension).
I will present techniques which help reduce the complexity of this infinite dimensional sampling problem. This is supported by theoretical analysis and numerical simulations.
Sarah James (University of Adelaide) - Modelling intensive care units using quasi-birth-and-death processes
October 21, 2019
When: 14:00
Where: F3.20 (KdVI)
An intensive care unit (ICU) is a crucial and limited resource in a hospital which is affected by uncertainty and
variability, and often operates close to capacity. Queueing theory has been used to model the bed occupancy in ICUs for the last 21 years, with a particular focus on using M/M/· and M/P H/· queueing models. These queueing models assume that the arrival process (patient arrivals) is a Poisson process, the service times (length of stay) follow an exponential or Phase-Type distribution, and most crucially that the arrival process and service times are independent of each other. However, Varney et al. showed that there is some dependence structure between the arrival process and the service times in an ICU. Without independence between the arrival process and service times, standard queueing models become invalid.
We aim to provide a more principled approach to modelling bed occupancy in ICUs using quasi-birth-and-death processes (QBDs). By allowing the phases of the arrival process and the service times to interact with each other, QBDs provide the flexibility to model a queueing system with dependence between the arrival process and the service times. In this talk, we describe the approaches we have taken to develop model-fitting procedures for QBD models, with a particular focus on fitting suitable QBD models to data from an intensive care unit.
Sarah James, University of Adelaide, Australia, sarah.james@adelaide.edu.au
Nigel Bean, University of Adelaide, Australia, nigel.bean@adelaide.edu.au
Jono Tuke, University of Adelaide, Australia, simon.tuke@adelaide.edu.au
Arijit Chakrabarty (ISI Kolkata) - Asymptotic behaviour of high Gaussian minima
April 10, 2019
When: 14:00
Where: F1.15 (KdVI)
Abstract: We investigate what happens when an entire sample path of a Gaussian process on a compact interval lies above a high level. Specifically, we determine the precise asymptotic probability of such an event, the extent to which the high level is exceeded, the conditional shape of the process above the high level, and the location of the minimum of the process given that the sample path is above a high level. The method of answering the questions are different for smooth and non-smooth processes. The former case is analyzed in much more details than the latter.
This is a joint work with Gennady Samorodnitsky and Zhixin Wu.

Cornelia Wichelhaus (TU Darmstadt) - Nonparametric Estimation in Stochastic Networks
March 21, 2019
When: 11:00 (on a Friday!)
Where: F3.20 (KdVI)
Abstract: Stochastic networks are interacting systems of nodes with service stations between which customers move in order to receive service. The talk addresses nonparametric estimation problems for these systems in discrete time. In particular we are interested in estimating the service time distributions at the nodes based on incomplete observations of the systems. We assume that we are only able to observe the external arrivals and departures of customers. We propose two estimation approaches. The first one is based on the construction of a so-called sequence of differences and the second utilizes the structure of cross-covariance functions between specific stochastic processes of the network. Both methods lead to deconvolution problems which we solve explicitly and which lead to consistent estimators. As main properties we show functional central limit theorems for the estimators in appropriate discrete spaces.
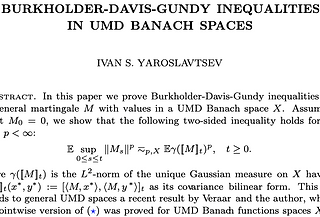
Ivan Yaroslavtsev (TU Delft) - Burkholder--Davis--Gundy inequalities and stochastic integration in UMD Banach spaces
February 20, 2019
When: 15:00
Where: F1.15 (KdVI)
Abstract: In this talk we will present Burkholder--Davis--Gundy inequalities for general UMD Banach space-valued martingales. Namely, we will show that for any UMD Banach space X, for any X-valued martingale M with M_0=0, and for any 1 \leq p < infty:
E sup_{0 \leq s \leq t} ||M_s||^p \eqsim_{p, X} E gamma([M]_t)^p, t \geq 0,
where [M]_t is the covariation bilinear form of M defined on X* x X* by
[M]_t(x*, y*) = [<M,x*>,<M, y*>]_t, for x*, y* in X*,
and gamma([M]_t) is the L2-norm of a Gaussian measure on X having [M]_t as its covariance bilinear form.
As a consequence we will extend the theory of vector-valued stochastic integration with respect to a cylindrical Brownian motion by van Neerven, Veraar, and Weis, to the full generality.

Siva Athreya (ISI Bangalore) - Respondent--Driven Sampling and Random Graph Convergence
February 04, 2019
When: 11:00
Where: F1.15 (KdVI)
Abstract: We consider certain respondent-driven sampling procedures on dense and sparse graphs. Under the assumption that the sequence of the vertex-sets is ergodic we understand possible limiting graphs. In the dense regime they can be expressed in terms of the original dense graph via a transformation related to the invariant measure of the ergodic sequence. In the sparse regime by a specific clumping procedure of the sampled vertices we construct a sequence of sparse graphs which converge to the governing graphon in the cut-metric.